LITERATURE REVIEW
1.2 General Principles of Earthquake Resistant Buildings
frame 3-3
frame 4-4
frame 5-5
frame 6-6
Storey drift in any storey due lateral force shall not exceed 0.004 times the storey height
5.3 Flexural Reinforcement

{If you are unable to see all details in google document just download it an run it on MS office of your system it will be clear}
Earthquake is a phenomenon that devastates the entire humankind. During such an event the
casualties are found to be in a large number because of the poorly constructed structures in
which we reside in. The major cause were found to be the weak beam-column joints, soft
ground storeys, improper and inadequate detailing, complex plan configuration and many more
by Prof C.V.R MURTY’S many research papers and publishes. IIT K people have done a decent
job in the preparation of examples on Earthquake resistant design of buildings. And also EERC
HYD people have found some critical reasons behind the collapse of buildings. The stiffness
variation and improper detailing are the reasons in common among all. Indian codes as well as
Euro codes laid recommendations for the design. The problems: stiffness variation and
improper detailing had been taken into consideration in the task. It is always the smart
buildings which perform better than the sturdiest one during an earthquake.
CHAPTER-1 DESIGN
According to IS: 456-2000, there are three methods for design of RC, Pre-stressed and Steel Structures which are as followed:
a) Working Stress Method
b) Ultimate Load method
c) Limit State Method(this method is followed in this report)
1.1 Limit State Method
In this method, all relevant limit states are considered in design to ensure an adequate degree of safety and serviceability. According to this method, the structure can achieve two different types of limit states:
a) Limit State of collapse
b) Limit state of serviceability
1.1.1 Limit State of Collapse
This limit may corresponds to Maximum load carrying capacity. Four different types of behavior of structure are:
a) Flexure
b) Compression
c) Shear
d) Torsion
1.1.2 Limit State of Serviceability
This limit state corresponds to development of excessive deformation. Three different types of behavior of structure in this state are:
a) Deflection
b) Cracking
c) Vibration
1.2 General Principles of Earthquake Resistant Buildings
The most happening principles to be considered for the design of earthquake –resistant structures are discussed as followed:
a) Design Basis Earthquake
b) Pseudo-Static Earthquake
c) Components of Acceleration
d) Increase in Permissible Stresses
e) Increase in Allowable Bearing Capacity
f) Horizontal and Vertical Inertia Forces
g) Resonance
h) Base Shear
CHAPTER-2 BUILDING PLANNING
2.1 Plan for G+2 Residential Building
The following two storey building is an RCC building with 18.35m length and 11.29m width. Floor to floor height from ground floor is 2m and for the other remaining stories, height is 3m (Total height 11m).
2.1.1 Orientation
After having selected the site, the next step is proper orientation of building. Orientation means proper placement of rooms in relation to sun, wind, rain, topography and outlook and at the same time providing a convenient access both to the street and back yard.
The factors that affect orientation most are as follows.
ü Solar heat
ü Wind direction
ü Humidity
ü Rain fall
ü Intensity of wind site condition
ü Lightings and ventilation
Based on it, arrangement of rooms are given as followed:
Table 2.1 Aspects of Room
S. No
|
Room
|
Aspect
|
1
|
Bed
|
NW – W - SE
|
2
|
Kitchen
|
E & rarely NE
|
3
|
Dinning
|
SE – S – SW
|
4
|
Drawing
|
SE – S – SW - W
|
5
|
Reading
|
N – NW
|
6
|
Store
|
NW – N – NE
|
Based on these factors, the plan layout is as shown below:
Building Plan
The building plan involves series of steps to achieve a proper detailed design of the building. The steps involved in this process are:
2.2 Grid Line Marking
The orientation of the columns is done in such a way to utilize the complete capacity of them. In general the depth of the column is placed along the longer direction to provide proper stability to structure.
2.3 Slab Numbering
2.4 Structural Details
Based on the Thumb-Rules which are in IS 456-2000, the details of Building are given as below in table 2.2.
Table 2.2 Structural Details
Total Length in X direction
|
11.29m
|
Total length in Y direction
|
18.35m
|
Total Height
|
8m
|
Live Load(Typical floor)
|
3 KN/m2
|
Live load ( Terrace)
|
1.5 KN/m2
|
Floor Finish(Typical Floor)
|
1 KN/m2
|
Water Proofing(Terrace)
|
1.2 KN/m2
|
Location
|
Guwahati
|
Zone no.
|
V
|
Z : Zone Factor
|
0.36
|
I : Importance Factor
|
1
|
R : Response Reduction Factor (OMRF)
|
3
|
Column Dimension
|
0.3x0.4m
|
Beam Dimension
|
0.23x0.3m
|
Slab Thickness
|
0.12m
|
External wall Thickness
|
0.23m
|
Internal, Parapet, Gallery wall Thickness
|
0.12m
|
Concrete Grade
|
M20
|
Steel Grade
|
Fe415
|
2.5 Gravity Load Calculations
The gravity loads are considered as per IS: 875. The different loads are considered as followed:
Table 2.3 Assumed Loads on Structures (IS 875 (Part I & II))
Live Load (Typical Floor)
|
3 KN/m2
|
Live Load (Terrace)
|
1.5 KN/m2
|
Floor Finish (Typical Floor)
|
1 KN/m2
|
Water Proofing (Terrace)
|
1.2 KN/m2
|
Table 2.4: Gravity Loads on Slab
Type of load
|
Name of load
|
Typical floor
|
Terrace
|
Units
|
Dead load
|
Self-Weight
|
3
|
3
|
KN/m2
|
Floor Finish
|
1
|
1
|
KN/m2
| |
Water Proofing
|
-
|
1.2
|
KN/m2
| |
Total
|
4
|
5.2
|
KN/m2
| |
Live load
|
3
|
1.5
|
KN/m2
|
2.6 Transfer of Loads from Slabs to Beams
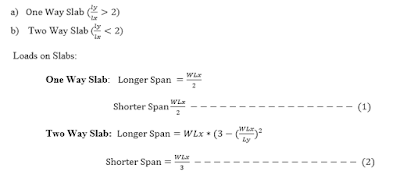
The gravity loads are transferred from slabs to beams, beams to columns, columns to foundations and foundations to soil. The loads coming from slabs to beams are transferred based on yield line theory. There are two types of slabs:
Table 2.5 Load Distribution of Slab
SLAB DESIGN
| |||||
Slab Name
|
Llonger
|
Lshorter
|
Ll/Ls
|
Type
| |
S1
|
5
|
4
|
1.25
|
Two Way
| |
S2
|
4
|
3
|
1.33
|
Two Way
| |
S3
|
5
|
4
|
1.25
|
Two Way
| |
S4
|
5
|
3
|
1.67
|
Two Way
| |
S5
|
3
|
3
|
1.00
|
Two Way
| |
S6
|
5
|
3
|
1.67
|
Two Way
| |
S7
|
6
|
5
|
1.20
|
Two Way
| |
S8
|
6
|
3
|
2.00
|
Two Way
| |
S9
|
6
|
5
|
1.20
|
Two Way
| |
S10
|
5
|
3
|
1.67
|
Two Way
| |
S11
|
3
|
3
|
1.00
|
Two Way
| |
S12
|
5
|
3
|
1.67
|
Two Way
| |
S13
|
5
|
4
|
1.25
|
Two Way
| |
S14
|
4
|
3
|
1.33
|
Two Way
| |
S15
|
5
|
4
|
1.25
|
Two Way
| |
S16
|
3
|
2
|
1.50
|
Two Way
| |
S17
|
5
|
2
|
2.50
|
One Way
| |
Slab Name
|
Llonger
|
Lshorter
|
Dead Load(kN/m)
|
Live Load(kN/m)
| ||||||
Typical Floor
|
Terrace
|
Typical Floor
|
Terrace
| |||||||
(m)
|
(m)
|
Lshorter
|
Llonger
|
Lshorter
|
Llonger
|
Lshorter
|
Llonger
|
Lshorter
|
Llonger
| |
S1
|
5
|
4
|
5.33
|
12.91
|
6.93
|
16.78
|
4
|
9.68
|
2
|
4.84
|
S2
|
4
|
3
|
4.00
|
10.13
|
5.20
|
13.16
|
3
|
7.59
|
1.5
|
3.80
|
S3
|
5
|
4
|
5.33
|
12.91
|
6.93
|
16.78
|
4
|
9.68
|
2
|
4.84
|
S4
|
5
|
3
|
4.00
|
11.52
|
5.20
|
14.98
|
3
|
8.64
|
1.5
|
4.32
|
S5
|
3
|
3
|
4.00
|
4.00
|
5.20
|
5.20
|
3
|
3.00
|
1.5
|
1.5
|
S6
|
5
|
3
|
4.00
|
11.52
|
5.20
|
14.98
|
3
|
8.64
|
1.5
|
4.32
|
S7
|
6
|
5
|
6.67
|
15.65
|
8.67
|
20.34
|
5
|
11.74
|
2.5
|
5.87
|
S8
|
6
|
3
|
4.00
|
12.50
|
5.20
|
16.25
|
3
|
9.38
|
1.5
|
4.69
|
S9
|
6
|
5
|
6.67
|
15.65
|
8.67
|
20.34
|
5
|
11.74
|
2.5
|
5.87
|
S10
|
5
|
3
|
4.00
|
11.52
|
5.20
|
14.98
|
3
|
8.64
|
1.5
|
4.32
|
S11
|
3
|
3
|
4.00
|
4.00
|
5.20
|
5.20
|
3
|
3.00
|
1.5
|
1.50
|
S12
|
5
|
3
|
4.00
|
11.52
|
5.20
|
14.98
|
3
|
8.64
|
1.5
|
4.32
|
S13
|
5
|
4
|
5.33
|
12.91
|
6.93
|
16.78
|
4
|
9.68
|
2
|
4.84
|
S14
|
4
|
3
|
4.00
|
10.13
|
5.20
|
13.16
|
3
|
7.59
|
1.5
|
3.80
|
S15
|
5
|
4
|
5.33
|
12.91
|
6.93
|
16.78
|
4
|
9.68
|
2
|
4.84
|
S16
|
3
|
2
|
2.67
|
7.26
|
3.47
|
9.44
|
2
|
5.44
|
1
|
2.72
|
S17
|
5
|
2
|
4.00
|
5.20
|
3.00
|
1.50
| ||||
2.7 Wall Load Calculations
External Wall(230 mm thick )
|
12KN/m
|
Internal Wall(120mm thick)
|
6.25KN/m
|
Gallery Wall(120mm thick)
|
3KN/m
|
Parapet Wall(230mm thick)
|
4KN/m
|
Self-Weight of Beam (Load/unit weight)
|
1.725KN/m
|
table 2.6 Wall Load Calculations
2.8 Load Distribution by Yield Line Theory on Typical Floor
Load Distribution by Yield Line Theory on Top Floor
Total Loading on All Frames
frame 1-1
frame 2-2
frame 3-3
frame 4-4
frame 5-5
frame 6-6
frame 7-7
2.9 Analysis of 2D Frame Using Kani’s Method (For Frame A-A)
Out of all the classical methods of analysis of portal frame, this method is the easiest and approximate method. Though it is an iterative process, it is self-correcting. This method uses the following formulas:
Fixed End Moments:
Final End Moments:
CHAPTER-3 3D model SAP 2000
3.1 3D View of Building Plan
CHAPTER-4 LATERAL LOAD ANALYSIS
The primary purpose of all kinds of buildings is to resist gravity loads besides vertical loads caused by wind loads, blasting or earthquake also develop high stresses, produce sway movements or causes vibration. Therefore, it is important for a structure to resist against vertical loads together with gravity loads.
4.1 Methods for Determining of Design Lateral Loads
a) Equivalent Static Method
b) Dynamic Analysis
1) Response Spectrum Analysis
2) Time History Analysis
4.1.1 Equivalent Static Method
This Concept is most commonly used because it converts dynamic forces into equivalent static forces for finding out the maximum displacements induced in the structure. This equivalence is restricted to a single mode of vibration of the structure.
Step 1:
Preliminary data from IS 1893:
- Building Height is less than 90m hence; Equivalent Static Analysis (ESA) can be applied.
- Building is located in Guwahati in Zone V, so Zone Factor Z = 0.36(IS 1893).
- Building is resting on soft soil.
Step 2:
Table 4.1 Seismic Weight of Ground Floor
Components
|
Length (m)
|
Width (m)
|
Depth (m)
|
Density (kN/m3)
|
Numbers
|
Weight (kN)
| ||
Beam along Y
|
23.5
|
0.3
|
0.4
|
25
|
4
|
282
| ||
Beam along X
|
13.9
|
0.3
|
0.4
|
25
|
7
|
291.90
| ||
Column
|
2.5
|
0.3
|
0.4
|
25
|
28
|
210
| ||
External wall Load along X
|
27.8
|
0.23
|
1.35
|
20
|
1
|
172.64
| ||
External wall Load along Y
|
21.5
|
0.23
|
1.35
|
20
|
2
|
267.03
| ||
Internal wall Load along X
|
33.8
|
0.11
|
1.35
|
20
|
1
|
100.3 9
| ||
Internal wall Load along Y
|
37.1
|
0.11
|
1.35
|
20
|
1
|
110.19
| ||
Gallery wall load
|
10.6
|
0.11
|
1.25
|
20
|
1
|
29.15
| ||
Stair case Load
|
12.06
|
12.06
| ||||||
Stair case Live Load
|
10
|
10
| ||||||
25% OF live load
|
2.5
|
2.5
| ||||||
Total Seismic Load(25% Live Load)
|
1477.85
| |||||||
Table 4.2 Seismic Weight of Typical Floor
Components
|
Length (m)
|
Width (m)
|
Depth (m)
|
Density (kN/m3)
|
Numbers
|
Weight (kN)
| |
Slab 1 Load
|
8.6
|
2
|
0.18
|
25
|
1
|
77.4
| |
Slab 2 Load
|
13.9
|
21.3
|
0.18
|
25
|
1
|
1332.32
| |
Beam along Y
|
23.5
|
0.3
|
0.4
|
25
|
4
|
282
| |
Beam along X
|
13.9
|
0.3
|
0.4
|
25
|
7
|
291.90
| |
Column
|
2.5
|
0.3
|
0.4
|
25
|
28
|
210
| |
Floor Slab 1
|
8.6
|
2
|
0
|
1
|
1
|
17.2
| |
Floor Slab 2
|
13.9
|
21.3
|
0
|
1
|
1
|
296.07
| |
External wall Load along X
|
27.8
|
0.23
|
2.7
|
20
|
1
|
345.28
| |
External wall Load along Y
|
21.5
|
0.23
|
2.7
|
20
|
2
|
534.06
| |
Internal wall Load along X
|
33.8
|
0.11
|
2.7
|
20
|
1
|
200.77
| |
Internal wall Load along Y
|
37.1
|
0.11
|
2.7
|
20
|
1
|
220.37
| |
Gallery wall Load
|
10.6
|
0.11
|
1.25
|
20
|
1
|
29.15
| |
Stair case Load
|
15
| ||||||
3851.52
| |||||||
Live load Slab 1
|
8.6
|
2
|
0
|
3
|
1
|
51.6
| |
Live load Slab 2
|
13.9
|
21.3
|
0
|
3
|
1
|
888.21
| |
Stair case Live Load
|
5.7
| ||||||
25%live load
|
236.38
| ||||||
Total Seismic Load (25% Live Load)
|
4087.89
| ||||||
Table 4.3 Seismic Weight of Top Floor
Components
|
Length (m)
|
Width (m)
|
Depth (m)
|
Density (kN/m3)
|
Numbers
|
Weight (kN)
| ||
Slab 1 Load
|
8.6
|
2
|
0.18
|
25
|
1
|
77.4
| ||
Slab 2 Load
|
13.9
|
23.5
|
0.18
|
25
|
1
|
1469.93
| ||
Beam along Y
|
23.5
|
0.3
|
0.4
|
25
|
4
|
282
| ||
Beam along X
|
23.9
|
0.3
|
0.4
|
25
|
7
|
501.90
| ||
Column
|
1.5
|
0.3
|
0.4
|
25
|
28
|
126
| ||
External wall Load along X
|
27.8
|
0.23
|
1.35
|
20
|
1
|
172.64
| ||
External wall Load along Y
|
21.5
|
0.23
|
1.35
|
20
|
2
|
267.03
| ||
Internal wall Load along X
|
33.8
|
0.11
|
1.35
|
20
|
1
|
100.39
| ||
Internal wall Load along Y
|
37.1
|
0.11
|
1.35
|
20
|
1
|
110.19
| ||
Stair case Load
|
2
|
30
| ||||||
Total Seismic Load
|
3137.47
| |||||||
Step 3:
Fundamental Natural Period
Of a moment resisting frame building without brick infill panels may be estimated as
4.1.2 Distribution of Base Shear To Storey Shear
Step 7:
Table 4.4 Distribution of Base Shear to Storey Shear
STOREY
|
Wi (kN)
|
H (m)
|
Wi*Hi2
|
Qi (kN)
| ||
3
|
3137.47
|
8
|
200798
|
849
| ||
2
|
4087.89
|
5
|
102197
|
432
| ||
1
|
1477.85
|
2
|
5911
|
25
| ||
SUMMATION
|
308907
|
1305
| ||||
Seismic Force at Floor Levels along X-direction (all units in KN)
4.1.3 Distribution of Lateral Storey Load to each Frame
Distribution of Lateral Load to each frame is in proportion to stiffness of each frame.
Table 4.5 Distribution of Storey Shear to Frame Shear along X-direction
Frame
|
Stiffness (kN/m)
|
Dis.Factor
|
I-Storey
|
II-Storey
|
III-Storey
|
Grid 1
|
10000
|
0.14
|
3.50
|
60.47
|
118.80
|
Grid 2
|
10000
|
0.14
|
3.50
|
60.47
|
118.80
|
Grid 3
|
10000
|
0.14
|
3.50
|
60.47
|
118.80
|
Grid 4
|
10000
|
0.14
|
3.50
|
60.47
|
118.80
|
Grid 5
|
10000
|
0.14
|
3.50
|
60.47
|
118.80
|
Grid 6
|
10000
|
0.14
|
3.50
|
60.47
|
118.80
|
Grid 7
|
10000
|
0.14
|
3.50
|
60.47
|
118.80
|
Table 4.6 Distribution of Storey Shear to Frame Shear along Y-direction
Frame
|
Stiffness (kN/m)
|
Dis.Factor
|
I-Storey
|
II-Storey
|
III-Storey
|
Grid A
|
25000
|
0.25
|
6.25
|
107.98
|
212.1
|
Grid B
|
25000
|
0.25
|
6.25
|
107.98
|
212.1
|
Grid C
|
25000
|
0.25
|
6.25
|
107.98
|
212.1
|
Grid D
|
25000
|
0.25
|
6.25
|
107.98
|
212.1
|
4.2 Centre of Mass and Centre of Stiffness Calculations
4.2.1 Centre of Mass
Where Wi =Weight of components (Slab, Beam, Wall, Column and Staircase)
Component
|
CG(X) (m)
|
CG(Y) (m)
|
Breadth (m)
|
Length (m)
|
Mass (Kg)
|
M x X
|
M x Y
|
Slab 1
|
9.56
|
22.3
|
2
|
8.53
|
7677
|
73392.12
|
171197.1
|
Slab 2
|
6.91
|
10.65
|
21.3
|
13.83
|
132560.55
|
915993.4
|
1411769.86
|
Beam 1
|
6.91
|
0
|
0.3
|
13.83
|
4149.00
|
28669.59
|
0
|
Beam 2
|
6.91
|
4.3
|
0.3
|
13.83
|
4149.00
|
28669.59
|
17840.70
|
Beam 3
|
6.91
|
7.53
|
0.3
|
13.83
|
4149.00
|
28669.59
|
31241.97
|
Beam 4
|
6.91
|
13.76
|
0.3
|
13.83
|
4149.00
|
28669.59
|
57090.24
|
Beam 5
|
6.91
|
17.0
|
0.3
|
13.83
|
4149.00
|
28669.59
|
70491.51
|
Beam 6
|
6.91
|
21.29
|
0.3
|
13.83
|
4149.00
|
28669.59
|
88332.21
|
Beam 7
|
6.91
|
23.3
|
0.3
|
13.83
|
4149.00
|
28669.59
|
96671.70
|
Beam 8
|
0
|
11.65
|
0.3
|
23.3
|
6990.00
|
0
|
81433.50
|
Beam 9
|
5.3
|
11.65
|
0.3
|
23.3
|
6990.00
|
37047.00
|
81433.50
|
Beam 10
|
8.53
|
11.65
|
0.3
|
23.3
|
6990.00
|
59624.70
|
81433.50
|
Beam 11
|
13.83
|
11.65
|
0.3
|
23.3
|
6990.00
|
96671.70
|
81433.50
|
Wall 1
|
9.56
|
23.3
|
0.11
|
8.53
|
2345.75
|
22425.37
|
54655.98
|
Wall 2
|
13.83
|
22.3
|
0.11
|
2
|
550
|
7606.5
|
12265
|
Wall 3
|
6.91
|
21.3
|
0.23
|
13.83
|
19085.4
|
131880.11
|
406519.02
|
Wall 4
|
2.65
|
17.0
|
0.11
|
5.3
|
3498
|
9269.7
|
59431.02
|
Wall 5
|
11.18
|
17.0
|
0.11
|
5.3
|
3498
|
39107.64
|
59431.02
|
Wall 6
|
2.65
|
13.76
|
0.11
|
5.3
|
3498
|
9269.7
|
48132.48
|
Wall 7
|
2.65
|
7.53
|
0.11
|
5.3
|
3498
|
9269.7
|
26339.94
|
Wall 8
|
11.18
|
7.53
|
0.11
|
5.3
|
3498
|
39107.64
|
26339.94
|
Wall 9
|
2.65
|
4.3
|
0.11
|
5.3
|
3498
|
9269.7
|
15041.4
|
Wall 10
|
6.91
|
0
|
0.23
|
13.18
|
18188.4
|
125681.84
|
0
|
Wall 11
|
1
|
11.76
|
0.11
|
2
|
1320
|
1320
|
15523.2
|
Wall 12
|
0
|
21.3
|
0.23
|
21.3
|
29394
|
0
|
626092.2
|
Wall 13
|
2
|
14.26
|
0.11
|
5
|
3300
|
6600
|
47058
|
Wall 14
|
5.3
|
19.14
|
0.11
|
4.3
|
2838
|
15041.4
|
54319.32
|
Wall 15
|
5.3
|
10.64
|
0.11
|
6.23
|
4111.8
|
21792.54
|
43749.55
|
Wall 16
|
5.3
|
2.15
|
0.11
|
4.3
|
2838
|
15041.4
|
6101.7
|
Wall 17
|
8.53
|
12.26
|
0.11
|
9.43
|
6223.8
|
53089.01
|
76303.79
|
Wall 18
|
8.53
|
3.76
|
0.11
|
7.53
|
4969.8
|
42392.4
|
18686.45
|
Wall 19
|
13.83
|
10.65
|
0.23
|
21.3
|
29394
|
406519.02
|
313046.1
|
Stair case
|
5.3
|
22.3
|
2
|
0.16
|
96
|
508.8
|
2140.8
|
342884
|
2348908.5
|
4181546.2
|
Table 4.7 Centre of Mass Calculation
Centre of Mass
Table 4.8 Centre of Stiffness Calculation
Component
|
CG(X) (m)
|
CG(Y) (m)
|
Width (m)
|
Depth (m)
|
Leangth (m)
|
Kx
|
Ky
|
Kx * X
|
Ky * Y
|
Column 1
|
0
|
0
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
0
|
0
|
Column 2
|
5.3
|
0
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
84.27
|
0
|
Column 3
|
8.6
|
0
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
136.75
|
0
|
Column 4
|
13.9
|
0
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
221.02
|
0
|
Column 5
|
0
|
4.3
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
0
|
38.46
|
Column 6
|
5.3
|
4.3
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
84.27
|
38.46
|
Column 7
|
8.6
|
4.3
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
136.75
|
38.46
|
Column 8
|
13.9
|
4.3
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
221.02
|
38.46
|
Column 9
|
0
|
7.6
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
0.00
|
68.0
|
Column 10
|
5.3
|
7.6
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
84.27
|
67.98
|
Column 11
|
8.6
|
7.6
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
136.75
|
67.98
|
Column 12
|
13.9
|
7.6
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
221.02
|
67.98
|
Column 13
|
0
|
13.9
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
0.00
|
124.33
|
Column 14
|
5.3
|
13.9
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
84.27
|
124.33
|
Column 15
|
8.6
|
13.9
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
136.75
|
124.33
|
Column 16
|
13.9
|
13.9
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
221.02
|
124.3
|
Column 17
|
0
|
17.2
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
0.00
|
153.84
|
Column 18
|
5.3
|
17.2
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
84.27
|
153.84
|
Column 19
|
8.6
|
17.2
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
136.75
|
153.84
|
Column 20
|
13.9
|
17.2
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
221.02
|
153.84
|
column 21
|
0
|
21.5
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
0.00
|
192.30
|
column 22
|
5.3
|
21.5
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
84.27
|
192.30
|
column 23
|
8.6
|
21.5
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
136.75
|
192.3
|
column 24
|
13.9
|
21.5
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
221.02
|
192.30
|
column 25
|
0
|
23.5
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
0.00
|
210.19
|
column 26
|
5.3
|
23.5
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
84.27
|
210.19
|
column 27
|
8.6
|
23.5
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
136.75
|
210.19
|
column 28
|
13.9
|
23.5
|
0.3
|
0.4
|
3
|
15.90
|
8.94
|
221.02
|
210.19
|
Table 4.9 Final Lateral Force in Frames along X direction
Frame
|
Storey 1
|
Storey 2
|
Storey 3
|
1
|
3.94
|
68.16
|
133.91
|
2
|
3.80
|
65.66
|
128.99
|
3
|
3.69
|
63.70
|
125.15
|
4
|
3.54
|
61.08
|
120.00
|
5
|
3.65
|
63.09
|
123.96
|
6
|
3.80
|
65.66
|
128.99
|
7
|
3.87
|
66.88
|
131.39
|
A
|
0.63
|
10.83
|
21.28
|
B
|
0.15
|
2.59
|
5.10
|
C
|
0.12
|
2.14
|
4.20
|
D
|
0.58
|
10.07
|
19.78
|
Table 4.10 Final Lateral Force in Frames along Y direction
Frame
|
Storey 1
|
Storey 2
|
Storey 3
|
1
|
0.44
|
7.69
|
15.11
|
2
|
0.30
|
5.19
|
10.19
|
3
|
0.19
|
3.23
|
6.35
|
4
|
0.04
|
0.61
|
1.20
|
5
|
0.15
|
2.62
|
5.16
|
6
|
0.30
|
5.19
|
10.19
|
7
|
0.37
|
6.41
|
12.59
|
A
|
6.88
|
118.81
|
233.38
|
B
|
6.40
|
110.57
|
217.20
|
C
|
6.37
|
110.12
|
216.30
|
D
|
6.83
|
118.05
|
231.88
|
. 4.4: Storey Drift
Table 4.11 Storey Drift Calculations in X- direction
STOREY
|
HEIGHT(mm)
|
DISP.(mm)
|
STOREY DRIFT
|
MAX. DRIFT
|
LIMIT
|
II FLOOR
|
3000
|
18.8
|
6.9
|
12
|
OK
|
I FLOOR
|
3000
|
11.9
|
9.1
|
12
|
OK
|
PLINTH
|
2000
|
2.8
|
2.8
|
8
|
OK
|
Table 4.12 Storey Drift Calculations in Y- direction
STOREY
|
HEIGHT(mm)
|
DISP.(mm)
|
STOREY DRIFT
|
MAX. DRIFT
|
LIMIT
|
II FLOOR
|
3000
|
17
|
6
|
12
|
OK
|
I FLOOR
|
3000
|
11
|
8.4
|
12
|
OK
|
PLINTH
|
2000
|
2.6
|
2.6
|
8
|
OK
|
Hence the building is safe.
CHAPTER-5 DESIGN OF SLAB
Example Solution for Slab1:
Table 5.1 Assumed Data of Depth
ASSUMED DEPTH
| |
Depth
|
120mm
|
Diameter of Bar
|
10mm
|
Cover
|
15mm
|
Support Width
|
300mm
|
Effective Depth Along X
|
100mm
|
Effective Depth Along Y
|
90mm
|
Effective Depth:
spacing required= 185.83mm
5.1.1 Loading on Slab
Table 5.3 Loading on Slab (all units are kn/m2)
Self-Weight of slab
| |
Floor finish
|
1
|
Live load(For typical floor)
|
3
|
Live load (For terrace)
|
1.5
|
Factored Dead load
| |
Factored live load
|
Table 5.4 Effective Length and Bending Moment Coefficients
Slab Name
|
Llonger
|
Lshorter
|
Depth of Slab (D) (mm)
|
ɸ of Bar (mm)
|
Cover(C) (mm)
|
Support Width (mm)
|
Ll/Ls
|
Type
|
S1
|
5
|
4
|
120
|
10
|
15
|
230
|
1.25
|
Two Way
|
S2
|
4
|
3
|
120
|
10
|
15
|
230
|
1.33
|
Two Way
|
S3
|
5
|
4
|
120
|
10
|
15
|
230
|
1.25
|
Two Way
|
S4
|
5
|
3
|
120
|
10
|
15
|
230
|
1.67
|
Two Way
|
S5
|
3
|
3
|
120
|
10
|
15
|
230
|
1.00
|
Two Way
|
S6
|
5
|
3
|
120
|
10
|
15
|
230
|
1.67
|
Two Way
|
S7
|
6
|
5
|
120
|
10
|
15
|
230
|
1.20
|
Two Way
|
S8
|
6
|
3
|
120
|
10
|
15
|
230
|
2.00
|
Two Way
|
S9
|
6
|
5
|
120
|
10
|
15
|
230
|
1.20
|
Two Way
|
S10
|
5
|
3
|
120
|
10
|
15
|
230
|
1.67
|
Two Way
|
S11
|
3
|
3
|
120
|
10
|
15
|
230
|
1.00
|
Two Way
|
S12
|
5
|
3
|
120
|
10
|
15
|
230
|
1.67
|
Two Way
|
S13
|
5
|
4
|
120
|
10
|
15
|
230
|
1.25
|
Two Way
|
S14
|
4
|
3
|
120
|
10
|
15
|
230
|
1.33
|
Two Way
|
S15
|
5
|
4
|
120
|
10
|
15
|
230
|
1.25
|
Two Way
|
S16
|
3
|
2
|
120
|
10
|
15
|
230
|
1.50
|
Two Way
|
S17
|
5
|
2
|
120
|
10
|
15
|
230
|
2.50
|
One Way
|
Design Moments
Table 5.5 Design Moments according to IS 456
αx-
|
αx+
|
αy-
|
αy+
|
0.0854
|
0.064
|
0.047
|
0.035
|
0.078
|
0.06
|
0.037
|
0.028
|
0.085
|
0.064
|
0.047
|
0.035
|
0.057
|
0.044
|
0.037
|
0.028
|
0.051
|
0.039
|
0.032
|
0.024
|
0.057
|
0.044
|
0.037
|
0.028
|
0.044
|
0.033
|
0.037
|
0.028
|
0.043
|
0.032
|
0.047
|
0.035
|
0.044
|
0.033
|
0.037
|
0.028
|
0.044
|
0.033
|
0.037
|
0.028
|
0.043
|
0.032
|
0.032
|
0.024
|
0.044
|
0.033
|
0.037
|
0.028
|
0.067
|
0.051
|
0.037
|
0.028
|
0.07
|
0.058
|
0.047
|
0.035
|
for, Mx- =185.83mm
Mx+ = 256mm
My- =233 mm
My+ =314.81 mm
b) Spacing required should be minimum of the following conditions
1) Spacing Required
2) 3 Times Effective Depth
3) 300mm
For,
Table 5.6
Design moments
|
A
|
B
|
C
|
Spacing provided mm
|
Mx-
|
300
|
300
|
185.83
|
180
|
Mx+
|
300
|
300
|
256
|
250
|
My-
|
270
|
300
|
233
|
230
|
My+
|
270
|
300
|
314.81
|
270
|
Table 5.7 No. of bars :-
Desigm moments
|
No. of bars provoded
|
Ast.(prov.) mm2
|
Mx-
|
6
|
471.24
|
Mx+
|
4
|
314.16
|
My-
|
5
|
392.69
|
My+
|
4
|
314.16
|
Table 5.8 %Pt:-
Desigm moments
|
%Pt
|
Fs
|
Modification factor
|
Mx-
|
0.47
|
215.87
|
1.42
|
Mx+
|
0.31
|
235.03
|
1.56
|
My-
|
0.44
|
206.58
|
1.6
|
My+
|
0.35
|
191.14
|
1.78
|
5.9 Check for Effective Depth (Typical and Terrace)
Table 5.10 Check for deflection (Typical and Terrace)
Design moments
|
L/d max
|
l/d (prov.)
|
Check
|
Mx-
|
36.92
|
31.88
|
Safe
|
Mx+
|
40.56
|
31.88
|
Safe
|
My-
|
41.6
|
40.67
|
Safe
|
My+
|
46.28
|
40.67
|
Safe
|
5.5 Deflection Check
The vertical deflection limits may generally be assumed to be satisfied, provided that the span to depth ratios are not greater than the max span to depth ratio.
5.6 Slab Detailing
5.6.1 Flexure Reinforcement
Bottom steel obtained from moment Mux+ and Muy+ should be uniformly distributed across the middle strip in short and long span directions respectively and to be provided up to 0.251 of a continuous edge and 0.151 of a discontinuous edge. It is also recommended that alternate bars should extend fully into the support.
Top steel obtained from moment Mux- and Muy- should be provided up to a distance of 0.151 and alternate bars up to 0.31 on either side of continuous support. At discontinuous edge tension reinforcement equal to 50 percent of that provided at mid span in same direction should be provided extending over a length of 0.11.
5.6.2 Torsional Reinforcement
Torsional Reinforcement is required at the corners of rectangular slab panels whose edges are discontinuous, it can be provided in form of mesh at top and bottom. Mesh should extend beyond the edge over a distance not less than one-fifth of the shorter span.

{If you are unable to see all details in google document just download it an run it on MS office of your system it will be clear}






























No comments:
Post a Comment